In a typical Calculus 2 class, sequences and series are learned near the end of the semester. In my Calculus 2 class in Spring 2020, we started with polynomial approximations to functions on day 1 of class. Why?
Constructing a sequence of functions (in this case, polynomials) that converge to a function is one of the most important ideas in analysis.
Polynomial approximations to functions introduce and provide context for sequences and series.
Uniform and pointwise convergence of polynomial approximations to a function can be explored and understood through graphs and spreadsheets, thereby providing students a means for understanding convergence beyond mere symbol pushing.
Polynomial approximations give students a way to understand how the function keys on their calculators actually work.
Students have more energy for learning at the beginning of a semester than at the end.
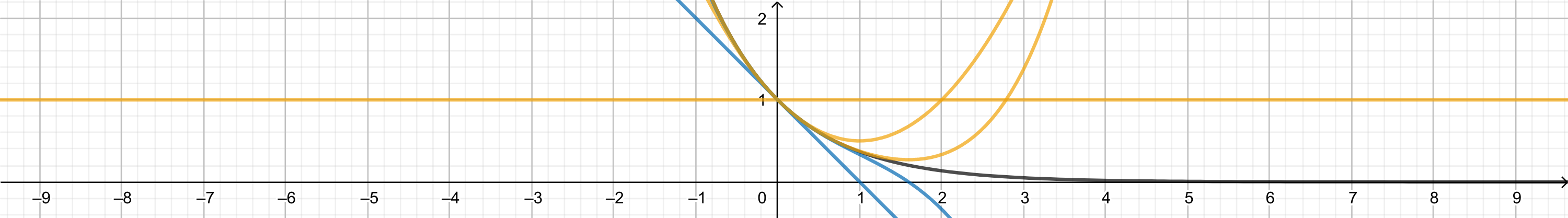
Instead of using Taylor’s theorem for constructing a polynomial approximation to a function via derivatives, we constructed polynomial approximations by integrating inequalities (inspired by Johnson). The basic idea is to bound a function $f$ above and below by polynomials $q$ and $p$ over an interval, integrate $p(t) \leq f(t) \leq q(t)$ over $\lbrack 0, x \rbrack$, and (if possible) solve the resulting inequalities for $f(x)$. This method works well when some antiderivative of $f$ is $f$ again (up to a constant), such as when $f(x)$ is $e^x$, $\sin(x)$, $\cos(x)$, $\sinh(x)$, and $\cosh(x)$. For example, I asked my students to do the following activities in class:
Show that if $f(t) \leq g(t)$ for all $t$ in $\lbrack a, b \rbrack$, then $\int_a^b f(t) \, dt \leq \int_a^b g(t) \, dt$.
Verify that $e^{-t} \leq 1$ for all $t \geq 0$. Then, integrate both sides of the inequality $e^{-t} \leq 1$ over $\lbrack 0, x \rbrack$ and solve the resulting inequality for $e^{-x}$ to get a degree $1$ polynomial approximation to $e^{-x}$. Then, repeat this process by integrating the result, $1-t \leq e^{-t} \leq 1$, over $\lbrack 0, x \rbrack$. Repeat this process many times and graph the new polynomial approximation at each step.
Write $p_n(x)$ for your degree $n$ polynomial approximation to $e^{-x}$. Solve the inequalities $p_5(x) \leq e^{-x} \leq p_4(x)$ for $| e^{-x} - p_4(x) | \leq ErrorBound(x)$. Using your error bound, find the largest positive $x$ such that the error between $p_4(x)$ and $e^{-x}$ is less than $0.0001$.
For more examples, see (Johnson). Some of the benefits to this approach are:
It is graphical, numerical, and symbolic, so it requires students to make connections among different viewpoints,
It reinforces the idea of an accumulation function from the Fundamental Theorem of Calculus,
The idea of convergence and error bounds are plain to see and easier to work with than Taylor’s remainder theorem, and
It’s fun!
References
Johnson, Wells. “Power Series Without Taylor’s Theorem.” The American Mathematical Monthly 91.6 (1984): 367-369. https://doi.org/10.1080⁄00029890.1984.11971434